Risk management - Probability density function PDF
Probability density function PDF
The problem with RISK assessment is precisely that, we are ASSESSING the risk we are NOT ABLE TO MEASURE IT accurately for each of the activities.
Many statistical analyses rely on measuring values for many items and plotting their frequency against the value. These values are usually related.
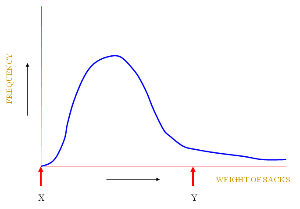
For example, if you were weighing sacks filled on a production line they would not all be a single value. If a few were weighed their weight would cover a range. If you extend the exercise to a 1000+ then you will get a curve of ‘weight of sacks’ against ‘frequency’ similar to above. The value that you expect the sacks to be (that which is most likely) will fall where the peak of the curve is.
The curve represents the likelihood (frequency) of any value for the weight of sack occurring.
From this process we can extract the RANGE (X to Y) of sack weights and it’s LIKELY (peak) value.
This graph is known as a PROBABILITY DENSITY FUNCTION (PDF) which we have seen earlier.
For a project trying to ascertain the possible values of COST in a single activity we have a slightly different approach. We know the range (set as the MINIMUM and MAXIMUM values) and we set the most LIKELY value. What we don’t know is how to predict the potential values in between. So we use a Probability Density Function or PDF to do so.
However, even the PDF can be simplified for most purposes. This is the 3 point estimate or ‘triangular distribution’.